Fate and Pathways of Organic and Inorganic Toxicant Pollutio
| 论文类型 | 基础研究 | 发表日期 | 2005-10-01 |
| 来源 | Macau Environment and City Development 2002 | ||
| 作者 | Zhishi,Wang | ||
| 摘要 | Macau Environment and City Development 2002 Fate and Pathways of Organic and Inorganic Toxicant Pollution in Macau Coastal Waters[2] | ||
Macau Environment and City Development 2002
Fate and Pathways of Organic and Inorganic Toxicant Pollution in Macau Coastal Waters[2]
Zhishi Wang
Faculty of Science and Technology, University of Macau, Macau
2. Physicochemical Modeling for Toxic Pollution in Water and Sediment Phases
Undoubtedly the field monitoring data can directly reflect the real world but a complex world. The toxic pollution in the coastal water sediments is a quite complicated problem involving various different pollution sources and pathways such as air/water exchange, surface adsorption and absorption, suspended particulates settling and transfer together with toxicants attached, resuspension of surface sediments, as well as long distance suspended solids transport in the tide flow field of the coastal waters. Only depending upon the field monitoring study, one cannot clearly understand the pollution fate and pathways. One of the reasons is that contaminated waters and sediments contain a mixture of aliphatic and aromatic organic compounds, each differing in its reactivity, solubility, toxicity, volatility, mineral surface affinity, and biodegradability. Another reason is that the suspended particulate matter and the sediment in natural systems are of a mixture containing phytoplankton, detritus, and clays and silts with different organic carbon contents and wide ranges of particle sizes. These different adsorbents do not behave independently. The accessibility of certain sorption reservoirs is probably influenced by the presence of others, and depends on the structure of the particles. The third reason may be that the hydrophobic organic compounds partitioning between suspended and solution phases is not always at equilibrium in water column. The field monitoring data are just reflecting the apparent consequence of interactions among these complicated processes. To clarify the fate and pathways of so many toxicant pollutions (various persistent organic species and heavy metals), physicochemical modeling for these processes and pollutants is essentially needed so as to imitate the reality by stressing those aspects that are assumed to be important and omitting all properties considered to be nonessential.
Bioavailability and ecotoxicology of the persistent toxicants (inorganic and organic) are closely related to their physicochemical transformation and partitioning between the overlying water phase and the suspended solid phase as well as the surface sediment phase. At present the laboratory assessment studies in the process mechanisms are still the most important tools to asses the impacts of these trace species on aquatic ecosystems of the coastal waters. The fundamental understanding of the process mechanisms is based on the chemical equilibrium concept, i.e., the equilibrium models for partitioning between the water and the sediment phases. In order to verify the validation of the laboratory-established models, the field monitoring for typical pollutants and sediments is necessary. Sometimes, considerations of the process kinetics are indispensable to extrapolate the laboratory study output to the actual cases.
2.1. Modeling for Water-Solid Phase Partition of Organic Compounds
Examining field monitoring data in Table 2 for various P-PAHs contents in surface sediment samples at different sites, one can see that the contents are only related to specific compounds in spite of different sites with different sediment particulates. The concentrations of different PAHs compounds in the sediment particulates vary in certain manner from the PAHs with low rings and low molecular weights to the high rings and high molecular weights. In order to insight the phase partitioning and distribution between pore water and sediment particulates, the partitioning coefficient Kd is defined for a single compound (e.g., pyrene) to measure the compound phase distribution between pore water and sediment particles:
Kd = Cs/Cw 1)
Where Cs - concentration of the compound partitioning to the particulate phase, ng/g; Cw - concentration of the compound in pore water, ng/l.
Chemically, the persistent organic pollutants in natural water environment are mostly of neutral and nonpolar organic compounds constructed primarily from carbon, hydrogen and halogen (e.g., Cl) atoms such as PAHs compounds. Most natural minerals such as sediment particulates are polar and expose a combination of hydroxyl- and oxy-moieties to their exterior [16]. Naturally, then these polar surfaces strongly favor interactions which allow them to form hydrogen bonds with polar water molecules, and unfavor directly adsorbing the nonpolar organic compound molecules such as pyrene and other PAHs on the natural mineral surfaces. In other words, replacing the water molecules at such a surface by nonpolar organic molecules is unfavorable from an energetic point of view. On the other hand, penetration of neutral organic chemicals into any natural organic matter such as humic substances in sediments does not require displacement of tightly bound water molecules. In light of this discussion, we may not be too surprised to find that nonreactive, neutral organic chemicals like PAHs show greater solid-water distribution ratios (Kd ) for soils and sediments that contain high amounts of natural organic matter. The neutral and nonpolar organic compounds are absorbed in the natural organic matter attached with sediment particulates instead of directly adsorbing to the mineral surfaces of the sediment particles. Therefore, another partitioning coefficient Kom is defined to measure allocation of the neutral organic chemicals between pore water and the natural organic matter in the sediment:
Kom = Com/Cw 2)
Where Com - concentrations of the compound absorbing in the natural organic matter e.g., humic substances in the sediment particulates, ng/g. The relationship between Kd and Kom is given by
Kd = fomKom 3)
Where fom - fraction of the natural organic matter content in the sediment particulates, %. The organic matter-water partition coefficient Kom is solely of an organic matter‘s property with no difference to different sediment particles. It has been found that the Kom values for a single neutral nonpolar chemical sorbing to a variety of soils and sediments are almost the same [16]. The Kd values are solely related to the natural organic matter contents fom; the higher the fom value, the larger the Kd value will be. The Cs values should follow the same pattern if the Cw values do not change too much.
Table 4 presents the analytic results for mineral compositions of the sediment particulates at different sampling sites of the coastal waters. We can see that the organic carbon fractions foc are almost the same for different sites ranging from 1 - 1.27 %, and the organic matter fractions fom can be calculated by doubling the foc values. As a result, the Kd values for a single neutral PAH chemical e.g. naphthalene (Na) or phenanthrene (Ph) should be almost the same among these different sites. Referring to Table 2, one can see that the Cs values for a single PAH chemical are in the same level, indeed, no matter where it was sampled. For example, the monitored Na contents (Cs) among the different sampling sites are mostly in the limited range of 30-50 ng/g dw and for the Ph contents, the Cs values vary in limited range from 110 to 160 ng/g dw.
In a fashion analogous to sediment-water partitioning, we can envision the molecules of a given compound partitioning between the organic phase and the aqueous phase. This partitioning process is determined by the relative fugacity of the compound in each phase and at equilibrium may be described by a dimensionless equilibrium constant.
The equilibrium constant can be also named as n-octonal-water partition coefficient:
Kow = Co/Cw 4)
Table 4 Analytical Result for Mineral Composition of Sediments
φ
rsw
rsw1 (kg/l) qMC 1.27 2.54 40.4 48.2 2.2 0.7 0.94 iMC 1.35 2.7 36.3 51.6 2.2 0.7 0.94 7.34x10-5 sMC 1.09 2.2 44 49.5 2.2 0.7 0.94 mMC 1 2 32 54 2.2 0.7 0.94 nMC 1.01 2 42.9 46.9 2.2 0.7 0.94 cMC 1.29 2.58 35.5 58.1 2.2 0.7 0.94 jMC 1.15 2.3 26.7 67.3 2.2 0.7 0.94 eMC 1.25 2.5 32 61.5 2.2 0.7 0.94
Where Co - the concentration of the compound in the organic phase, ng/l. Partially because of the choices of early workers [16] and the special amorphous nature of C4 to C10 alcohols, but mostly because n-octonal is a reasonable surrogate for many kinds of natural and environmental organic solvents, the n-octonal-water partition constant for a single nonpolar organic chemical such as pyrene is used to generalize the compound partitioning between any organic phase and the aqueous phase. The n-octonal-water partitioning coefficient has been investigated extensively. Because the attachment of a neutral nonpolar organic chemical on sediment particles is essentially of absorption process of the compound to the natural organic matter in the sediments, there is certain relation between the Kom and the Kow for the single compound. An empirical equation has been established to quantify the relationship:
logKom = clogKow + d 5)
Where c and d are empirical parameters whose values depend upon different compound classes. Karichhoff [17] examined the relationship between Kom and Kow for a series of the neutral nonpolar compounds (aromatic hydrocarbons, chlorinated hydrocarbons, chliro-S-trazines, phenyl ureas) by compiling the relevant data from different sources, and found that generally the values of parameters c and d were almost invariable and c = 0.82 and d = 0.14 if the unit of Kom is l/kg, instead of l/g. Table 5 gives the values of the Kow for a series of P-PAHs compound including the 2-ring PAH Na, and the 3-ring PAHs Fl, An, Ph, and the 4-ring PAHs Py, Flu, BaA as well as the 5-ring compound BaP. The calculated Kom values based on Equation 5) are also given by that table. One can see that the higher the molecular weight and ring numbers of the compound, the larger the Kom value it has, which means that the neutral nonpolar PAH compounds with high ring numbers and high molecular weights are more favorable to absorb into the natural organic matter attached to the sediment particulates than low ring and low molecular weight compounds. Combining Equations 3) and 5), one can get the following equation:
log Kd = clogKom + d + logfom 6)
Table 5 Calculated Partition Coefficients of P-PAHs
MW - molecular weight of PAH compound; Csat - solubility of the compound
With it, one can calculate Kd values for these P-PAHs compounds which are also given in Table 5. Armed with such a Kd parameter for a case of interest, we may evaluate what fraction of a compound is in water phase fw in a volume containing both solids and water by
fw = CwVw/(CwVw + CsMs) 7)
Where Vw - volume of water in total volume Vt, l; Ms - solid mass in the same total volume, kg. So considering Equation 1), one have:
fw = 1/{1 + (Ms/Vw) Kd } = 1/(1 + r Kd ) 8)
Where r - solid mass concentration in the total volume, kg/l. For the surface sediment bed, the solid concentration can be expressed by the sediment bed porosity Φ, which is defined by pore water volume/total volume. That is
r = ρs(1 - Φ)/Φ 9)
Where ρs - density of the solid, kg/l. The mineral compositions of the sediment particulates in the sampling sites are similar referring to Table 4. There are two major minerals silica and clay in the sediment particulates. Generally the silica counts for about 40% while the clay for 55%. The density of the sediment can be calculated by the weighting summary of silica density and clay density, which is 2.2 kg/l.
The compacted sediment bed porosity is usually 0.2 to 0.4, depending upon the particle size distribution. The larger the particle sizes, the larger the bed porosity values are. However, the surface sediment bed is not completely compacted. And there is a strong tendency of resuspension of the surface sediment due to strong shear stresses at the water/sediment interface. These both lead to a high porosity of the surface sediment bed, i.e., 0.7 - 0.8. With Equation 9), one can calculate the r value of the surface sediments in the coastal waters, which equals 0.94 kg/l. Substituting the Kd values in Table 5 into Equation 8), the fw values for the P-PAHs species in Table 5 can be calculated. The calculation results show an interesting fact that most of the PAHs are partitioning into the sediment phase while in pore water the PAHs are almost undetectable (fw = 0) except the 2-ring PAH Na (fw = 0.05). The higher the ring number and molecular weight, the favorable the compound is to partition into the sediment phase.
In April 2001, the water samples were taken at the water depths of 0.5, 1.2, 3.5, 4.8, 5.5 and 6 meter (bottom), respectively along a water column at Porto Interio, aiming at analyzing partitioning of the persistent organic compounds (organochlorine pesticides DDTs and HCHs, and 16 US EPA TCL PAHs) between the bulk water phase and the suspended solid phase in the column with different water depths. The suspended solid concentrations at different depths of the column were measured to be 1.7, 17.9, 42.5, 54, 58.5 and 73.4 mg/l at the depths of 0.5, 1.2, 3.5, 4.8, 5.5 and 6 meters, respectively. Based on the Kd and r values of different compounds and different depths, the fw values were calculated by Equation 8). The calculation shows a fact just opposite to the case of water-sediment partition that most of the PAHs are partitioning to the water phase instead of the suspended solids, particularly for 2-ring P-PAH Na. The calculated fw values for Na are all equal to 1 at different column depths, which means that the Na pollutants are in dissolved phase instead of particulate phase. On the other hand, the calculated fw value at the bottom for the 5-ring P-PAH BaP is 0.66, that is, the aqueous BaP pollution only counts for 66% of the total pollution and there still remain 34% absorbing onto the suspended particulates. However, at the top of the column (0.5m depth) where the suspended solids was only 1.7 mg/l, the calculated BaP fw value is 0.99, that is most of the BaP species are in dissolved phase at that depth.
The field monitoring data also show the same trend, i.e., all the 2-ring PAHs were in dissolved phase at these depths while most of the 5-6 ring P-PAHs in suspended particulate phase, particularly at the bottom of the column. The 3-4 ring P-PAHs were detected in both phases and the dissolved phase partition fractions decreased with the depth. The 4-ring PAHs more favored the solid phase than the 3-ring P-PAHs (please refer to Figure 2).
In light of the above discussion, we can conclude that for a certain neutral nonpolar compound like Na or BaP, the dissolved and adsorbed (particulate) concentrations per total volume of a water column, Ca and Cp can be expressed as
Cd = fwCt 10)
Cp = (1 - fw) Ct 11)
Where Ct = Cd + Cp, representing the total compound concentration in the water column. For low ring and low molecular weight compound at top layer of the column, the Kd value is small and the r value is also small, fw—> 1 and Cd —> Ct and Cp —> 0, i.e., the dissolved phase partition is dominant. On the other hand, for high ring and high molecular weight at the bottom of the column (large Kd and r), fw —> 0 and Cp—> 1 and Cd —> 0, this means that the absorbing or particulate phase is dominant.
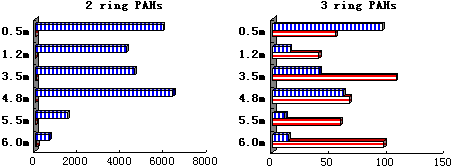
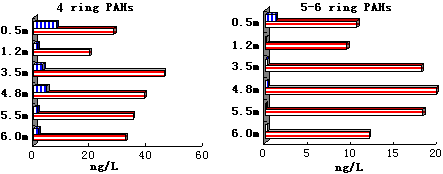
Figure 2. Field Monitoring Results for PAHs of Water-Suspended Solid Phases Partition in Water Column
(blue bar with vertical lines - dissolved phase; red bar with horizontal lines - suspended solid phase)
The conceptual model for the concentration of a persistent organic compound for which dissolved and particulate phases are both important can be established based on mass balance principle, i.e., the accumulation rate of the compound equals input rate subtracting output rate and interphase transfer loss rate as well as reaction decay rate. For the dissolved phase, we have
dCd/dt = Id - kwCd - kg(Cw - Ca/Kh) - krCd - Jtr 12)
Where Id - input rate of the compound in dissolved phase; kw - output rate constant and kw = 1/t and t - water column detention time; krCd - reaction decay rate by hydrolysis, photolysis, biodegradation of the compound. Because most of the toxic organic pollutants are chemically persistent, this term can be omitted. Jtr - the compound transfer rate from the dissolved to the absorbing phases per unit volume. The term kg(Cw - Ca/Kh) represents the compound transfer from the water phase to the air, where Kh is the water-air partitioning coefficient of the compound and Ca is the compound mass concentration in the air. The air/water phase exchange rate constant kg is determined by the compound molecular diffusion (Dw) and turbulent condition of the water/air boundary layers (e.g., wind speed at the water surface). For the sorbing or particulate phase, we have
dCp/dt = Ip - kwCp - ksCp - krCp + Jtr 13)
where Ip - input rate of the compound in sorbing phase; kwCp - output rate; krCp - reaction decay rate which can be omitted for most of the persistent organic pollutants. The term ksCp represents the sorbing compound transfer rate with the particle settling to the bottom where ks is the particle settling rate constant which is determined by particle Stoke‘s terminal velocity. Combining Equations 12) and 13), we have
dCt/dt = It + kgCa/Kh - kwCt - fwkgCt - (1 - fw)ksCt 14)
At steady state, i.e., dCt/dt = 0, we have
Ct = (It + kgCa/Kh)/(kw + kg + (1 - fw)ks) 15)
Where the air-water partitioning constant Kh is related to the Henry‘s constant by Kh‘/RT and the Henry‘s constant Kh‘ representing air/water phase equilibrium. For most of the persistent organic pollutants such as PAHs, it is generally quite small (less than 10-3). The air-water phase transfer rate constant kg is equal to vw/h, where vw is the compound transfer velocity in the viscous layer at the water surface and related to the compound molecular diffusion constant Dw. For most of the persistent organic pollutants Dw is also small (less than 10-6 cm2/s). The water-solid transfer rate constant ks is equal to vs/h where vs is the particle Stokes‘ terminal velocity. h is the water column depth.
2.2. Modeling for Heavy Metal Accumulation and Remobilization in Sediments
Table 3 gives the monitoring data for concentrations of Cd, Pb, Cr, and As species in the water phase and the sediment phase. A partitioning constant is defined to asses the accumulation of these heavy metals and the As species in the sediment as below:
Kd = [SoM]/[M] 16)
where [SoM] represents the concentration of the metal M in the sediment (μg/g) while [M] represents its concentration in water (mg/l). The measured partitioning constants for these species were in 7.6 - 20 l/g. With Equation 8), one can calculate the fw values for these species. For the water-sediment partitioning, the calculated values are all equal to 1, that is, all these pollutants accumulated in the sediment phase and undetectable in the pore water, just as the cases of the PAHs absorbing in sediments. For the water-suspended solid partitioning, the calculated values are in the narrow range near 0.5, i.e., the partitioning between the suspended and dissolved phases is just half to half.
The processes that govern the scavenging of trace metal elements by the particulate matter are complex, but generally can be considered to include adsorption, absorption, surface precipitation, and co-precipitation. In the oxic environment such as the oxygen-rich water column or the overlying suspended mud layers, the surface adsorption is dominant, and the allocation of the trace metals at water/solid interface can be estimated by the solid/water partitioning coefficients (Kd). The surface properties of the particles are an important key to understand interactions of trace metals and organic toxicants between dissolved and mineral phases in the coastal waters. The studies in particle surface characterization have suggested that an almost uniform coating of amorphous oxides or organic matter exists on the surfaces of the estuarine particles [18]. It is generally thought that iron and manganese oxides and organic matter coating are important phases for binding of trace metals in the sediment while clays can be only as a support for oxide and organic matter deposition or coating. The interactions of trace metals with the particle surfaces can be considered to be metal-ligand surface complex formations. The conceptual model can be established for interpreting the adsorption isotherms of trace metals at geothite oxide surface, based on the thermodynamic concept of surface coordination. The pH is a master parameter controlling the metal adsorption on oxide surfaces, and for a certain metal there is a special pH range where the adsorption dramatically increases.
Figures 3 gives experimental results of adsorption of Cu, Pb, Cd metal ions on goethite [10], which show that there was a narrow special pH range for each of the metal ions that an adsorption jump occurred, and the adsorption increased gradually with the solution pHs while the desorption occurred when the solution pHs decreased.
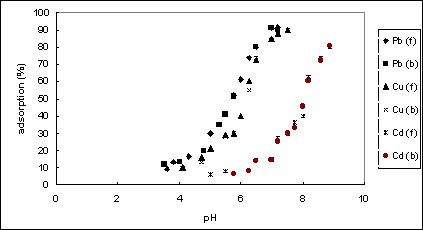
Figure 3. Adsorption (f) and desorption (b) of Pb, Cu, and Cd on geothite particles
This means that the adsorption is reversible with solution pHs. Viewed with chemical thermodynamics, and it can be considered as a process of metal-ligand surface complex formation, i.e.,
SºFeOH + M2+ÞSºoFeOM+ + H+ 17)
Where SoFeOH represents adsorption available site on goethite particle surfaces; SoFeOM+ is the particle surface site adsorbing metal ion M2+.
At equilibrium,
KS = ([SºFeOM+][H+])/([SºFeOH][M2+]) 18)
The log form of the above equation is given by
log([SºFeOM+]/[M2+]) = log KS + log[SºFeOH] + pH 19)
Combining Equations 16) and 18), one can have
log Kd = log KM + pH 20)
which means that the partitioning between water and sediment is controlled by pH, and Kd is proportionally related to the pore water pH. Equation 20) shows such a linear relationship between log Kd and pH. Figure 4 was drawn based on the experimental data in Figure 3. The linear relationships for these metal adsorption of goethite were verified by the experimental data.
The interruption of the ecological cycling at the interface does not only illustrate a decrease of pH in the pore water but also an accumulation of dissolved organic matter which is mostly constituted by organic acids such as acetic acid. These organic acids have a strong affinity to complexing with the heavy metals such as Cu in solution, which can re-mobilize these metals already adsorbed on the particles. Figure 5 gives the desorption experiment for the Cu/goethite system.
This experimental result reveals a competition of the surface metal-ligand complexing (SoFeOM+) and the solution complexing (ML) for the water-copper ions-organic acids- goethite system, which can be expressed by the following equation:
log{[ML]/[SºFeOM+]} = log K - log KS + log[HL-] - log[SºFeOH] 21)

Figure 4 Linear relationship between partition coefficient vs. pH
which shows that the more affinity the organic acid with the metal ion such as citric acid, the more re-mobilizing the metal is. In Equation 21), HL- represents the organic ligand, e.g., citric ligand and K is the solution complex formation equilibrium constant, and Log K = 0.80, -1.14, - 2.24, -1.51 for citric, tartaric, salicylic and phthatic ligand complex formation with Cu(II) in solution, respectively.

Figure 5 Copper adsorption on geothite particles in presence of citric(t),
tartaric - salicylic(g), phthatic(r) acids, respectively
Figure 6 gives the measurement of surface dissolution of goethite particles due to solution pH change and impact of organic acids (citric and tartaric acids). This shows that surface dissolution occurs at pH far from pHzpc of goethite; and presence of organic ligands largely promotes such a dissolution process.
These phenomena can also be explained using the conceptual model of metal-ligand complex formation at water/particle interface. The mechanisms of proton- and ligand-promoted surface dissolution of goethite involve two steps: a fast step of the surface complex formation (=FeOH2+ or =FeL-) and a subsequent slow step of release of ferric ions or ferric-ligand complexes from the goethite surface [19]. The metal release can also be caused due to surface-controlled dissolution of goethite in presence of organic acids.
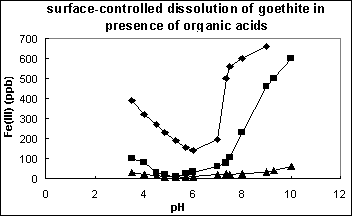
Figure 6 Surface-promoted dissolution of goethite in presence of organic acids
One of the mechanisms of metal re-mobilisation comes from the competitive effect between the metal-ligand complex formation in solution and the metal surface binding. The volatile fatty acids and humic acids that are the residues of organic matter degradation and decay as well as some synthetic organic compounds such as detergents, surfactants, tributyltin, etc. are all the available ligands for metals in the coastal waters leading to the metal re-mobilisation. Furthermore, some of the organic ligands such as acetic acids and salicylic acids can cause the surface dissolution of ferric oxide coated particles due to formation of solution complexes of these ligands with ferric ions in the lattice.
In this paper, the conceptual models are presented including the water-sediment partitioning model for persistent organic pollution in the Macau coastal waters and the surface complex formation model for heavy metal adsorption and desorption at water/sediment interfaces. All these models were established based on principles of organic and surface chemistry as well as the field observations. The model verification and calibration are further required by the designated laboratory experimental studies.
REFERENCES
1. J. Fu, Z. Wang, B. Mai, Y. Kang (2001) "Field monitoring of toxic organic pollution in the sediments of Pearl River estuary and its tributaries" Water Science and Technology, Vol 43, No 2, pp83-89
2. B. Mai, S. Wang, J. Fu, G. Sheng, P. Peng, Z. Wang, U. Tang (2001) "The pollution situation and sources of persistent organic compounds in sediments from Macao coastal waters" proceedings of Third Macau Symposium on Environment and City Development (Z. Wang, ed), University of Macau
3. H. Hong, L. Xu, L. Zhang (1995) "Environmental fate and chemistry of organic pollutants in the sediment of Xiamen and Victoria harbor" Marine Pollution Bulletin, Vol 31, No 4-12, pp229-236
4. D. Yuan, D. Yang, M. Chen, P. Xu, Y. Qian, J. Wang (2001) "Concentrations and distribution of polycyclic aromatic hydrocarbons and organo-chlorides in surface sediment of Xiamen western harbor and Mijiang estuary" Acta Scientiae Circumstantiae, Vol 21, No. 1, pp107-112
5. Z. Fang, R. Zhang, M. Huang (2001) "Concentrations and distribution of organochlorinated pesticides and PCBs in green-lipped mussels, Perna Viridis collected from the Pearl River estuarine zone" Acta Scientiae Circumstantiae, Vol 21, No. 1, pp113 -116
6. B. Mai, Z. Lin, G. Zhang, G. Sheng, Y. Min, J. Fu (2000) "Organic pollution in surface sediments of Pearl River estuary and its tributaries" Acta Scientiae Circumstantiae, Vol 20, No.21, pp192-197
7. B. Mai, Z. Lin, G. Zhang, G. Sheng, Y. Kang, P. Peng (2001) "Assessment of toxic organic pollution in sediments of Pearl River estuary" Environmental Science Research, Vol 14, No 1, pp 19-23
8. Y. Min, G. Zhang, S. Qi, G. Sheng, J. Fu, Z. Wang, U. Tang (2000) "Sedimentary history of heavy metal in Macao estuary, Southern China" proceedings of Second Macau Symposium on Environment and City Development (Z. Wang, ed), University of Macau
9. Z. Wang (1995) "An ecological assessment for water pollution in the estuary with high turbidity" Macau Science and Technology (L. Zhou ed), Macau Foundation press
10. Z. Wang, Q. Wang (2000) "Remobilization of metals ‘fixed‘ on goethite and kaolinite particles - a laboratory assessment of the heavy metal ‘second pollution‘ in estuary sediments" proceedings of Second Macau Symposium on Environment and City Development (Z. Wang, ed), University of Macau
11. G. Zhang, Y. Min, G. Sheng, J. Fu (1999) "Time trend of BHCs and DDTs in a sediment core in Macao estuary, Southern China" Marine Pollution Bulletin, Vol 39, No 1-12, pp325-329
12. J.E. Baker, S.J. Eisenreich (1990) "Concentrations and fluxes of polycyclic aromatic hydrocarbons and polychlorinated biphenyls across the air-water interface of Lake Superior" Environmental Science and Technology, Vol 24, pp342-352
13. J. Fu, Z. Wang, B. Mai (2002) "Identification of sources and pathways of persistent organic pollution (DDTs, PCBs, PAHs) in the sediment of coastal waters surrounding Macau" Annual Report for National Natural Science Foundation sponsored research project (No. 49972094)
14. Z. Wang, Y. Wang (1995) "Computer Modeling for pH Stability of the Anoxic Acidic Environment in the Sediment of the Macau Coastal Waters" in proceedings of International Conference on Education, Practice and Promotion of Computational Methods in Engineering Using Small Computer, 1 - 4 August, 1995, Macau
15. W. Camano, J. Hong, U. Forstner (1993) "Binding and mobilization of heavy metals in contaminated sediments affected by pH abd redox potential" Water Science and Technology, Vol 28, pp223-235
16. R.P. Schwarzenbach, P.W. Gschwend, D.M. Imboden (1993) "Chapter 11: Sorption, Solid-Aqueous Solution Exchange" Environmental Organic Chemistry, John Wiley & Sons Inc
17. S.W. Karickhoff (1981) "Semi-empirical estimation of sorption of hydrophobic pollutants on natural sediments and soils" Chemosphere, Vol 10, pp833-846
18. J.M. Martins et al. (1986) "Some recent developments in the characterization of estuarine particles" Environmental Science and Technology, Vol 18, pp83-92
19. Sulzberger et al. (1989) "Dissolution of Fe(III) (hydro) oxides in natural waters: laboratory assessment on kinetics controlled by surface coordination" Marine Chemistry, Vol 28, pp127-144
close
www.h2o-china.com论文搜索
月热点论文
论文投稿
很多时候您的文章总是无缘变成铅字。研究做到关键时,试验有了起色时,是不是想和同行探讨一下,工作中有了心得,您是不是很想与人分享,那么不要只是默默工作了,写下来吧!投稿时,请以附件形式发至 paper@h2o-china.com ,请注明论文投稿。一旦采用,我们会为您增加100枚金币。








